A Scalable Implicit Time-Stepping Method for the E3SM Ocean Model
Scientists have implemented the semi-implicit barotropic mode solver in the MPAS-O to allow faster and more stable simulation as a competitor of the existing split-explicit subcycling scheme. When implementing the semi-implicit method for any kind of numerical model, one of the most important issues is that the global collective process which requires all-to-all communication among cores should be called as small as possible to achieve better scalability. The barotropic mode solver uses a pipelined preconditioned bi-conjugate gradient method with the restricted additive Schwarz preconditioner, where the preconditioner plays as a role to accelerate the convergence of the iterative solver and therefore decrease the number of the global collective process up to 90%
This study shows the possibility of using the semi-implicit time-stepping algorithm in the MPAS-O through several test case experiments using a range of processor counts. The semi-implicit barotropic mode solver has better computational efficiency than the existing split-explicit subcycling scheme without degrading the accuracy. This new semi-implicit version of the MPAS-O can allow researchers to simulate their numerical experiments using the MPAS-O standalone as well as the integrated E3SM faster with more desirable stability.
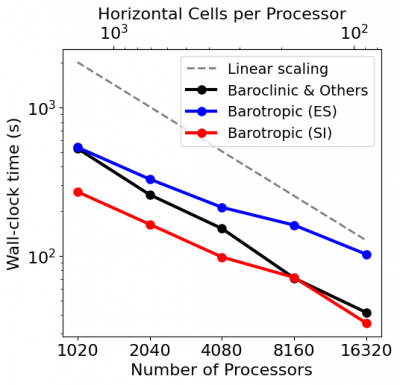
A scalable semi-implicit barotropic mode solver for the ocean component of the model for prediction across scales has been implemented as a competitor to an existing explicit-subcycling scheme to allow faster and more stable simulations while not sacrificing accuracy. The semi-implicit solver adopts the pipelined preconditioned bi-conjugate gradient stabilization algorithm as an iterative solver in conjunction with the restricted additive Schwarz preconditioner that accelerates the convergence rate of the iterative solver. The preconditioner is constructed from a linearized barotropic system that also reorders the system for optimal performance, while the semi-implicit solver deals with the fully nonlinear barotropic system that requires reassembly of the coefficient matrix for every time step. Several numerical experiments, from simple one-dimensional tests to three-dimensional real-world tests, demonstrate that the semi-implicit solver has almost the same accuracy and better parallel scalability compared with the existing scheme while allowing faster and more stable simulations. Furthermore, the semi-implicit solver accelerates the barotropic mode up to 2.9 times faster than the existing scheme on 16,320 processors, leading to an overall runtime speedup of 1.9.